X exponentiated by two plus negative Y raised to the power two ;How did you make exponents on the computer?The base is X;

Solved Quiz No 2 Expand The Following 1 X 9 12 2 X Y 9 3 X Y 1s 4 X Y 5 X Y 8
Expand (1/x+y/3)^2
Expand (1/x+y/3)^2-( 3 k)!Free math problem solver answers your algebra, geometry, trigonometry, calculus, and statistics homework questions with stepbystep explanations, just like a math tutor




Expand X 3 3 Expand 2 Y 3
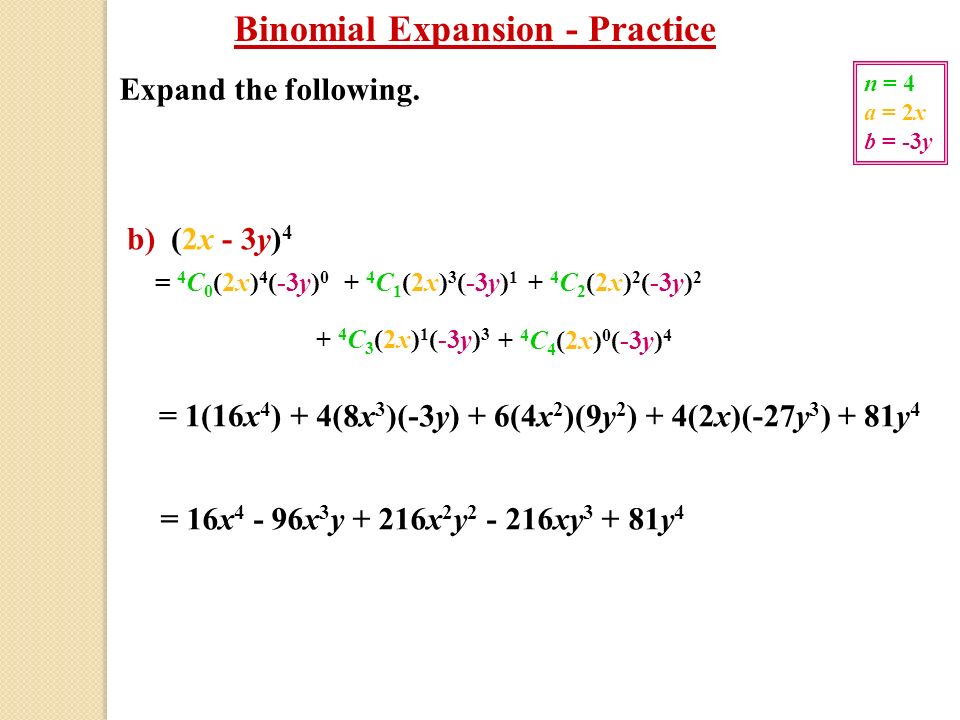
Algebra Expand using the Binomial Theorem (3xy)^3 (3x − y)3 ( 3 x y) 3 Use the binomial expansion theorem to find each term The binomial theorem states (ab)n = n ∑ k=0nCk⋅(an−kbk) ( a b) n = ∑ k = 0 n n C k ⋅ ( a n k b k) 3 ∑ k=0 3!⋅(x)3−k ⋅(−y)k ∑ k = 0 3 Result A sum containing 2 terms;
Algebra Factor x^3y^3 x3 − y3 x 3 y 3 Since both terms are perfect cubes, factor using the difference of cubes formula, a3 −b3 = (a−b)(a2 abb2) a 3 b 3 = ( a b) ( a 2 a b b 2) where a = x a = x and b = y b = y (x−y)(x2 xyy2) ( x y) ( x 2 x y y 2)Expand (xy)^3 (x y)3 ( x y) 3 Use the Binomial Theorem x3 3x2y3xy2 y3 x 3 3 x 2 y 3 x y 2 y 3The base is Y;
In elementary algebra, the binomial theorem describes the algebraic expansion of powers of a binomial According to the theorem, it is possible to expand the polynomial n into a sum involving terms of the form axbyc, where the exponents b and c are nonnegative integers with b c = n, and the coefficient a of each term is a specific positive integer depending on n and b For example,The second term of the sum is equal to a negative power; For expanding the equation we know the formula of cube of two number which is given below Now we will compare the given equation with the formula we will get the value of a and b which is Put these value in above equation and simplify it Now simplify the above expand form to get the answer Now further simplify the terms



Expand 3x Y 5 Using The Binomial Theorem Mathsgee Homework Help Exam Prep



How Do I Solve This Should I Expand The Equation I Found The Answer As 0 But Im Not Sure R Sat
Answer The expansion of (xy) 3 is x 3 y 3 3x 2 y 3xy 2 Let us see how to expand (xy) 3 Explanation The expression (xy) 3 can be written as, (xy)(xy)(xy) First simplify (xy)(xy) by binomial multiplication (xy)(xy) = x 2 2xy y 2 Now multiply (xy) with x 2 2xy y 2 (xy)(xy)(xy) = (xy)(x 2 2xy y 2) = x 3 2x 2 y xy 2 yx 2 2xy 2 y 3 = x 3 3x 2 y 3xy 2 yAlgebra Expand using the Binomial Theorem (xy)^3 (x − y)3 ( x y) 3 Use the binomial expansion theorem to find each term The binomial theorem states (ab)n = n ∑ k=0nCk⋅(an−kbk) ( a b) n = ∑ k = 0 n n C k ⋅ ( a n k b k) 3 ∑ k=0 3!Expand 2(x y) 3(x y) Equations Basic (Linear) Solve For Quadratic Solve by Factoring Completing the Square Quadratic Formula Rational



Solved Expand Each Binomial Using The Binomial Theorem 3x Chegg Com



A Quick And Efficient Way To Expand Binomials Ppt Download
The exponent is two;The first term of the sum is a power;Expand using the Binomial Theorem (x3)^3 (x 3)3 ( x 3) 3 Use the binomial expansion theorem to find each term The binomial theorem states (ab)n = n ∑ k=0nCk⋅(an−kbk) ( a b) n = ∑ k = 0 n n C k ⋅ ( a n k b k) 3 ∑ k=0 3!




Example 22 Ii Write The Cubes In Expanded Form 5p 3q 3
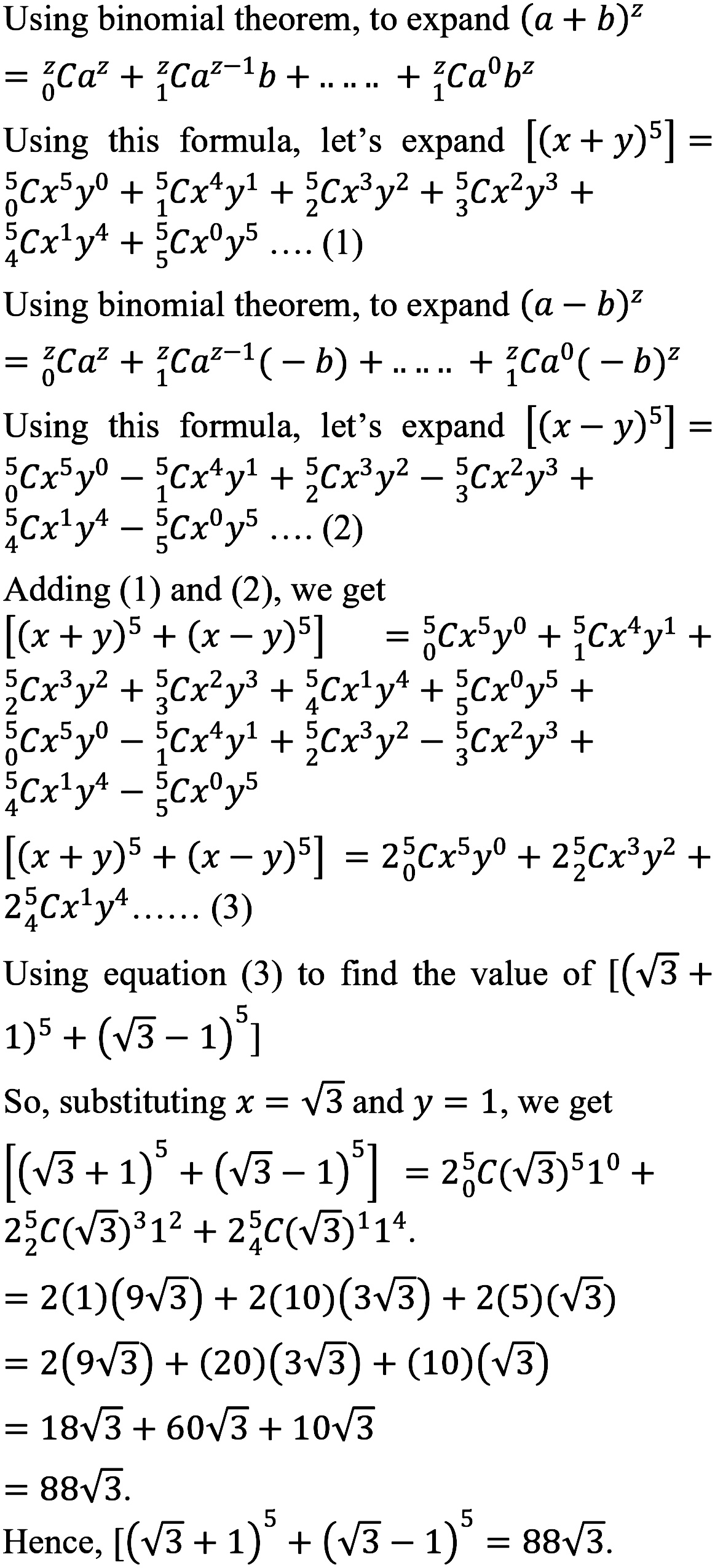



Using Binomial Theorem Expand X Y 5 X Y 5 And Hence Find The Value Of Sqrt 3 1 5 Sqrt 3 1 5 Snapsolve
It is clear that when $x=y$ we have $x^3y^3=0$ Then use long division to divide $x^3y^3$ by $xy$ and the result will be the equation on the right Another way would be to write $$\left(\frac{x}{y}\right)^3 1$$ Now we wish to find the zeros of this polynomialIn the case where x greatly exceeds y, the numerator is essentially 2 y ( 3 x) = 6 x y, and the denominator is approximately 2 x Therefore, the given expression is roughly 3 x 1 / 2 y, as claimedLearn about expand using our free math solver with stepbystep solutions



How To Expand X 2 3 Using Pascal S Triangle Quora




6 Expand C Xy 19 0 19 0 949
Expand (xy)^3 full pad » x^2 x^ {\msquare} \log_ {\msquare} \sqrt {\square} \nthroot \msquare {\square} \le \ge (xy)3= (x² 2xy y²)(xy) Then you FOIL (First, outer, inner, last) (xy)3 = (x² 2xy y²)(xy) = x2x 2xxy xy2 x2y 2xyy y2y and when you combine like terms = x3 3x2y 3xy2 y3 Upvote •8Downvote Comments •4 More Report Tyler C Thank you, Kristin! Explanation note that (x a)3 = x3 (a a a)x2 (aa aa aa)x a3 (x −1)3 → a = −1 ⇒ (x − 1)3 = x3 ( −1 − 1 − 1)x2 (1 1 1)x ( −1)3 = x3 −3x2 3x − 1




Left The Binary Tree Of The Expression Defined In The Codeblock 14 Download Scientific Diagram



How Do You Use The Binomial Series To Expand 2x Y 9 Socratic
⋅(3x)3−k ⋅(−y)k ∑ k = 0 3 ⋅ ( x) 3⋅(x)3−k ⋅(3)k ∑ k = 0 3 3!




Q Expand The Following Using The Suitable Identity Iv 0 1x 0 2y 3 Maths Polynomials Meritnation Com



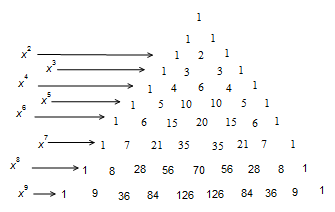
Pascal S Triangle And The Binomial Theorem X Y 0 1 X Y 1 1x 1y X Y 2 1x 2 2xy 1y 2 X Y 3 1x 3 3x 2 Y 3xy 2 1 Y 3 X Ppt Download
Expand (x2)^3 (x 2)3 ( x 2) 3 Use the Binomial Theorem x3 3x2 ⋅23x⋅ 22 23 x 3 3 x 2 ⋅ 2 3 x ⋅ 2 2 2 3 Simplify each term Tap for more steps Multiply 2 2 by 3 3 x 3 6 x 2 3 x ⋅ 2 2 2 3 x 3 6 x 2 3 x ⋅ 2 2 2 3 Raise 2 2 to the power of 2 2The exponent is two;
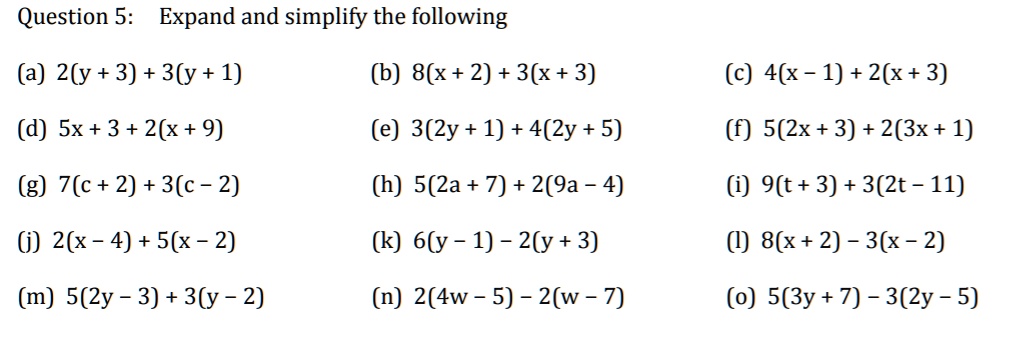



Solved Question 5 Expand And Simplify The Following A 2 Y 3 3ly 1 B 8 X 2 3 X 3 C 4x 1 2 X 3 D
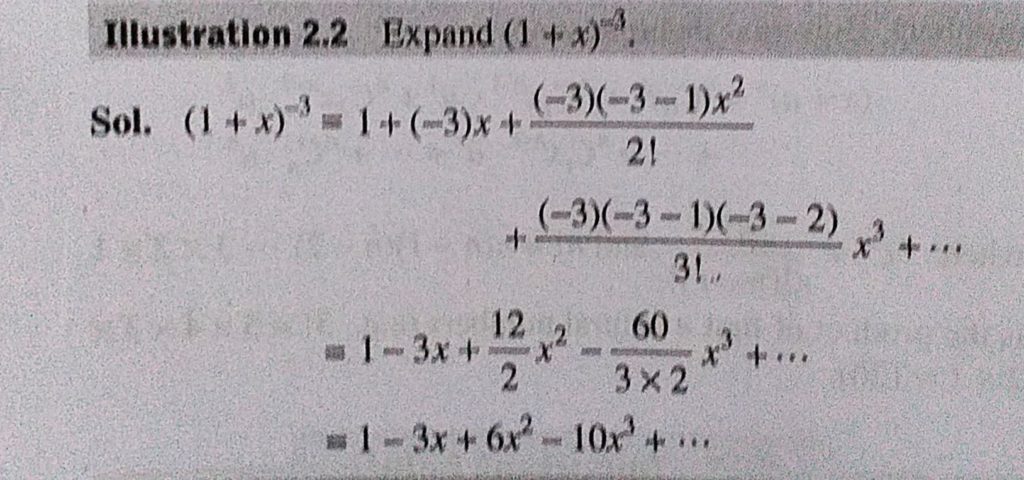



Expand 1 X 3 Sahay Lms




J23




Binomial Expansion Binomial Expansions Copyright C By Houghton Mifflin Company Inc All Rights Reserved 2 The Binomial Theorem Provides A Useful Method Ppt Download



Matthenes Com



X3 Y3 Formula




Ml Aggarwal Solutions For Class 9 Chapter 3 Expansions Download Free Pdf
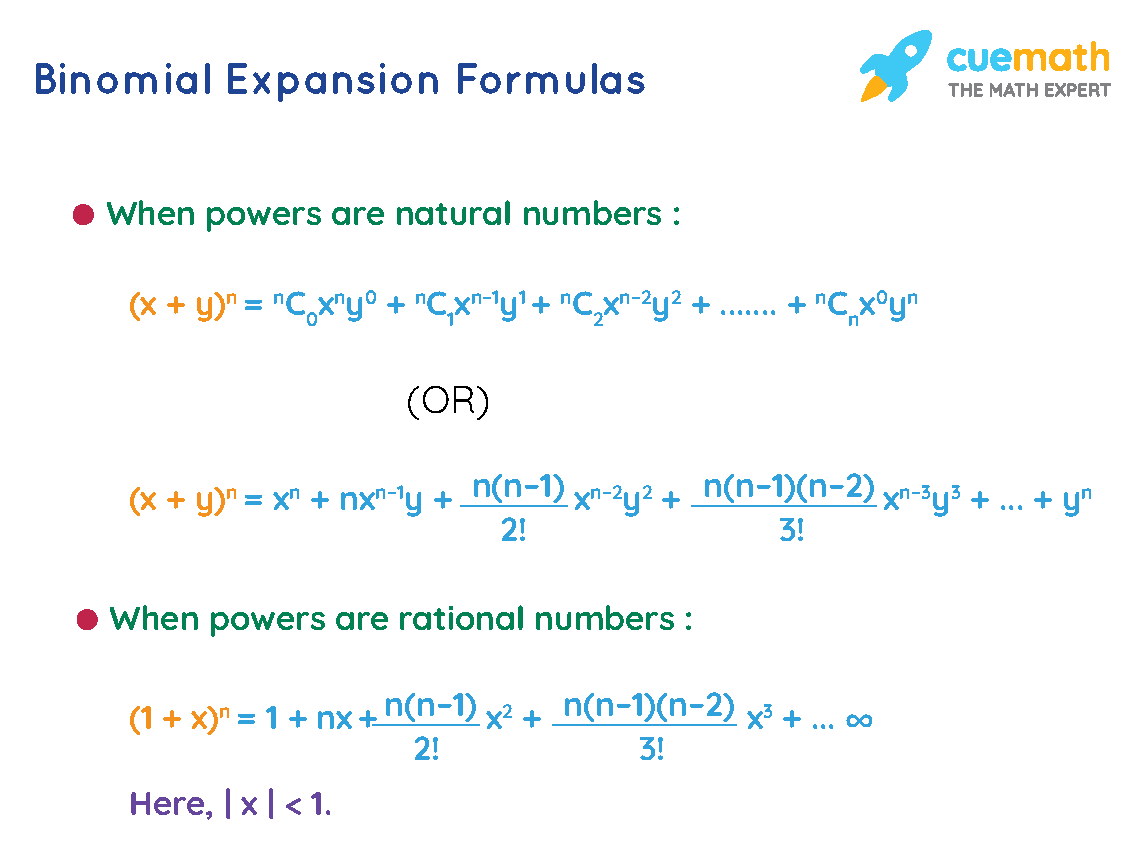



Binomial Expansion Formulas Derivation Examples



Techmat Vgtu Lt




Solved 18 Following Scripts Print Syms Xy F X Y 3 Expand F B Factor Syms X Y F X Y 3 Expand F B D Q Coursehigh Grades




Std 9 Expand X 1 Y Youtube
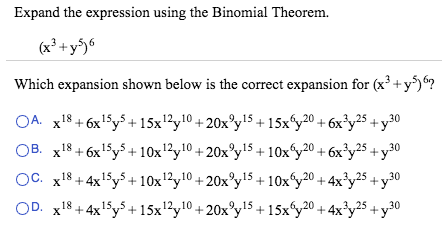



Solved Expand The Expression Using The Binomial Theorem Chegg Com




Write Each Of The Expanded Form 3x 2y 3



Solved Find The 5th Term In The Expansion 3x 2y 6 2 What Is The Sum Of The First 36 Terms In The Arithmetic Series 0 2 0 Course Hero



2



What Is The Answer Of X Y Quora
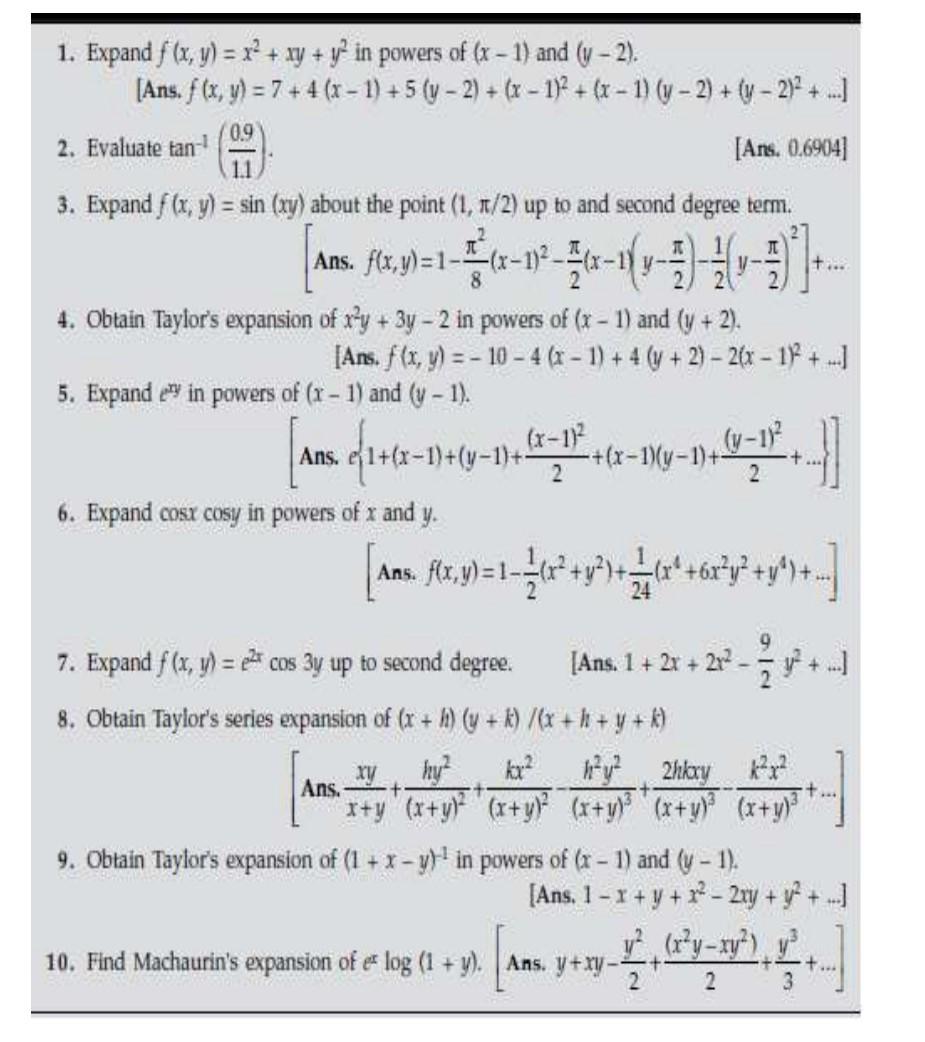



Solved 1 Expand F X Y X2 Xy Y2 In Powers Of X 1 Chegg Com
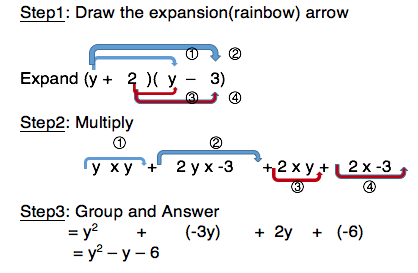



Themathbooklets S2t3 Expansion Of Linear Expressions




Expand Each Of The Following Using Suitable Identities




Online Tutoring On Maths Binomial Theorem




Example 22 I Write The Cubes In Expanded Form 3a 4b 3




1 Expand The Following A 2x Y B X 3y F R 2y D 2a Scholr
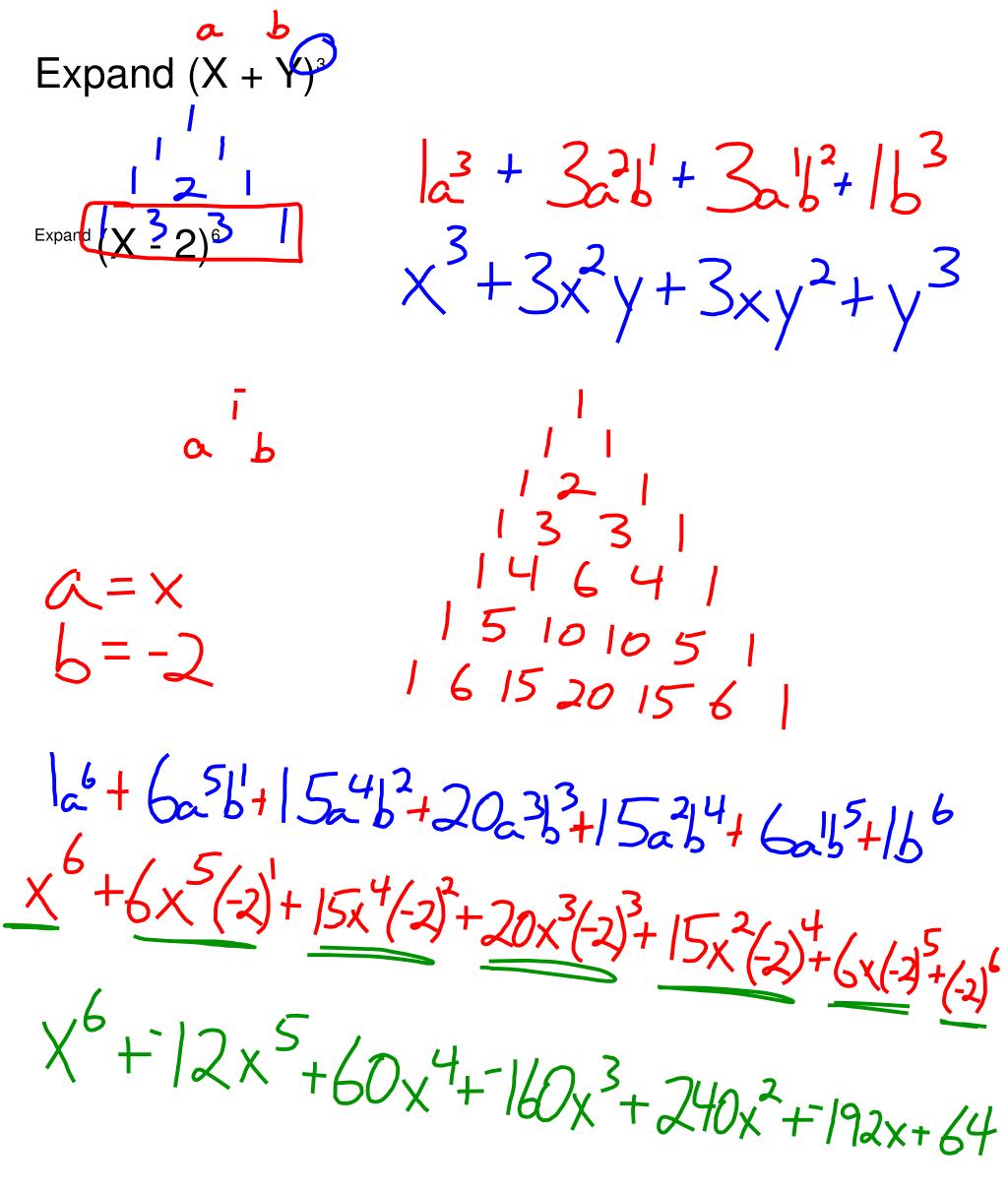



Ppt Algebra 2 C H 6 Notes Page 23 P 23 6 8 Pascal S Triangle And The Binomial Theorem Powerpoint Presentation Id




Binomial Theorem




1 X Y 3 3 Expand Brainly In




Expand Using Formula X Y 3 Brainly In
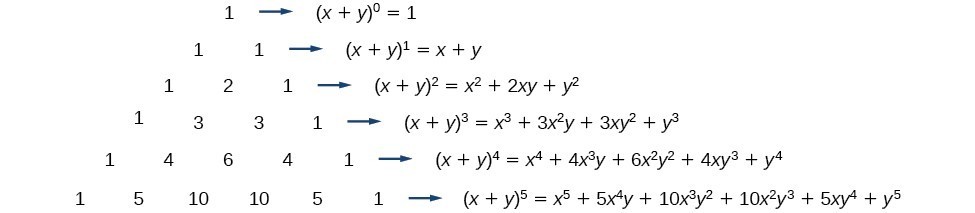



Using The Binomial Theorem College Algebra
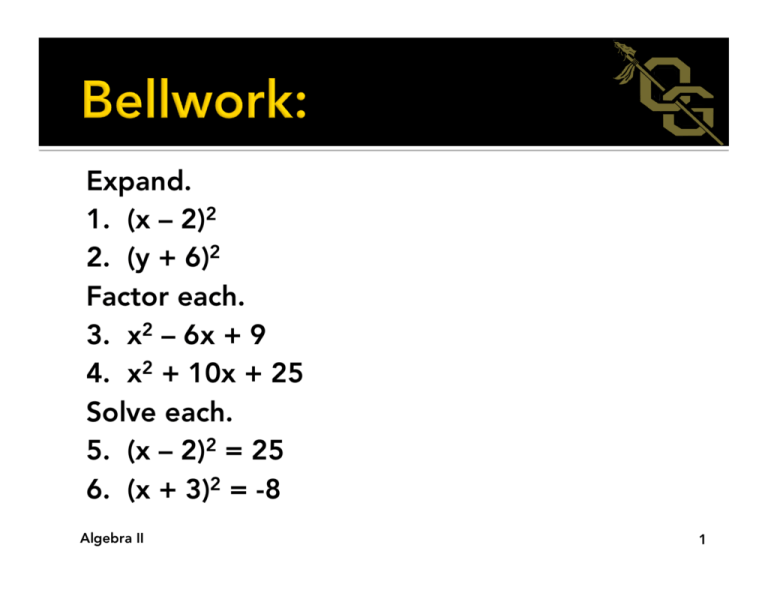



Expand 1 X 2 2 2 Y 6 2 Factor Each 3 X2 6x 9 4 X2 10x



Expand Log X2 Y3 Z4 Snapsolve
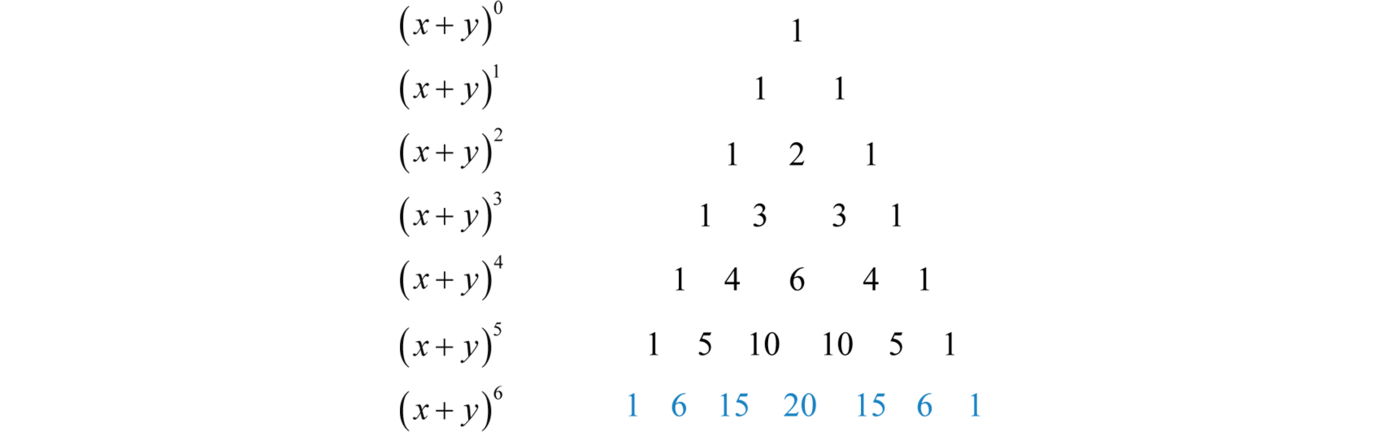



9 4 Binomial Theorem Mathematics Libretexts




Solved In Exercises 33 54 Use Either The Binomial Theorem Pascal S Triangle To Expand Each Binomial 33 X 1 34 X 2 4 35 X 1 5 36 1 X S 37



Using Binomial Theorem Expand Each Of The Following 3 X 3 Y 6 Sarthaks Econnect Largest Online Education Community
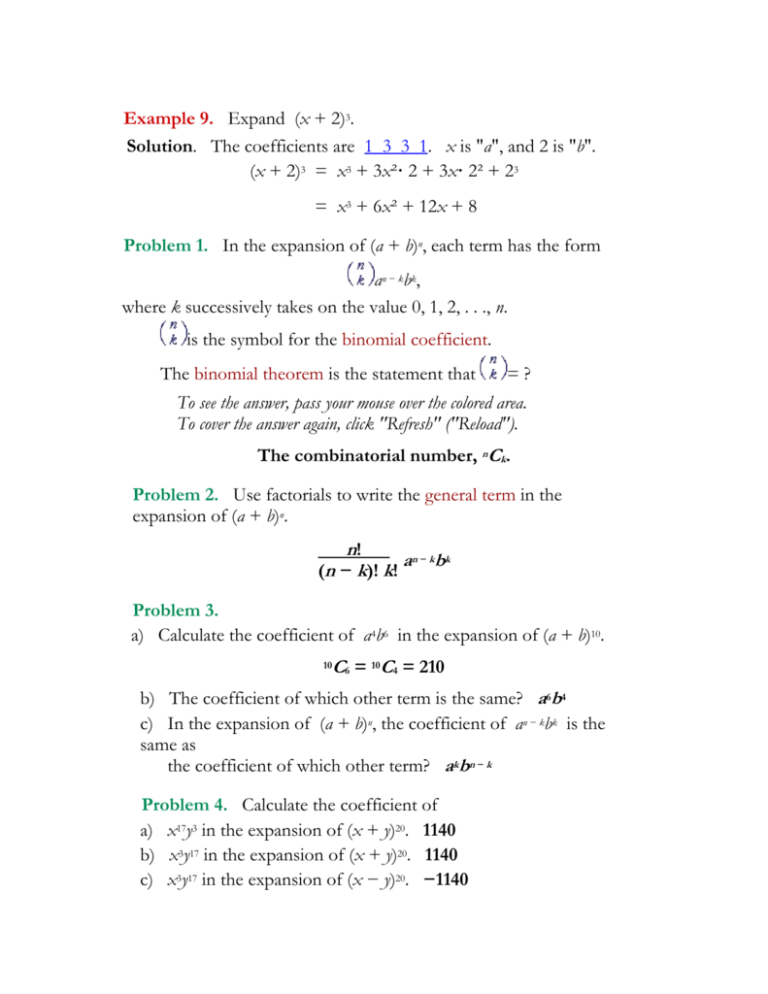



Alg2 March28 The Answers




Expand X 3 3 Expand 2 Y 3




Binomial Coefficients Best Way To Expand 2 X X 2 6 Mathematics Stack Exchange
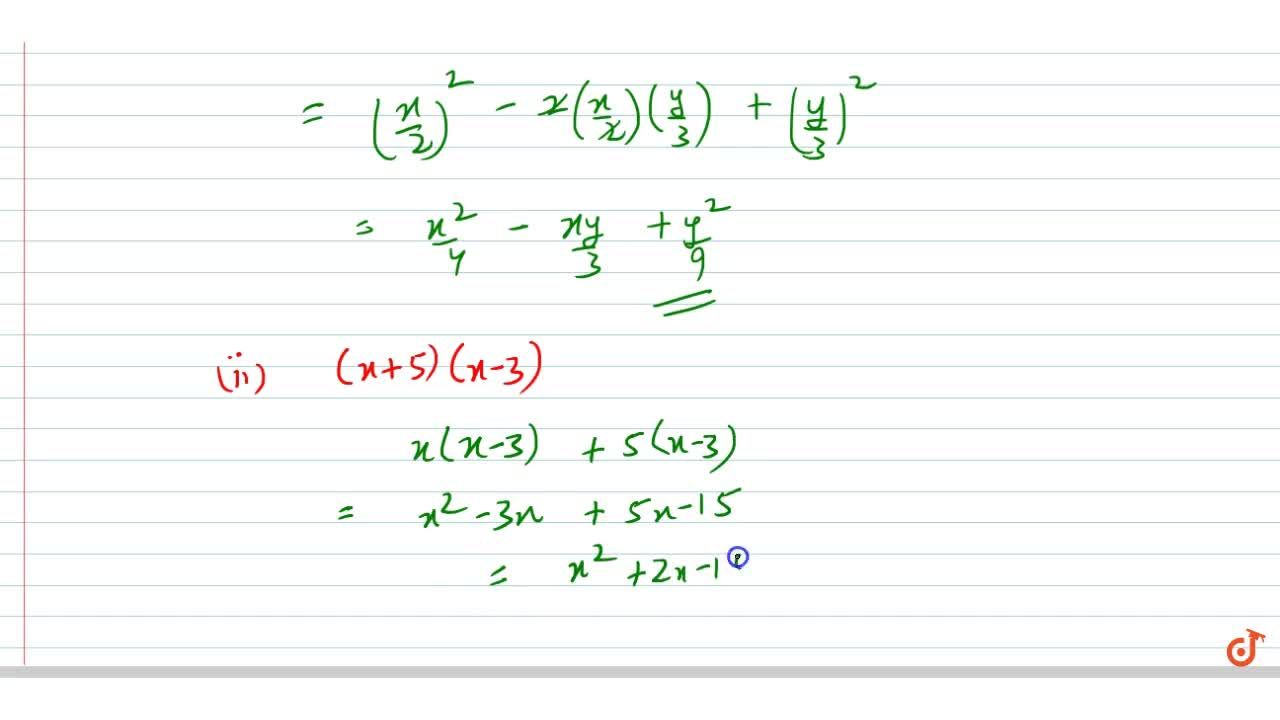



Expand Each Of The Following I X 2 Y 3 2 Ii X 5 X 3
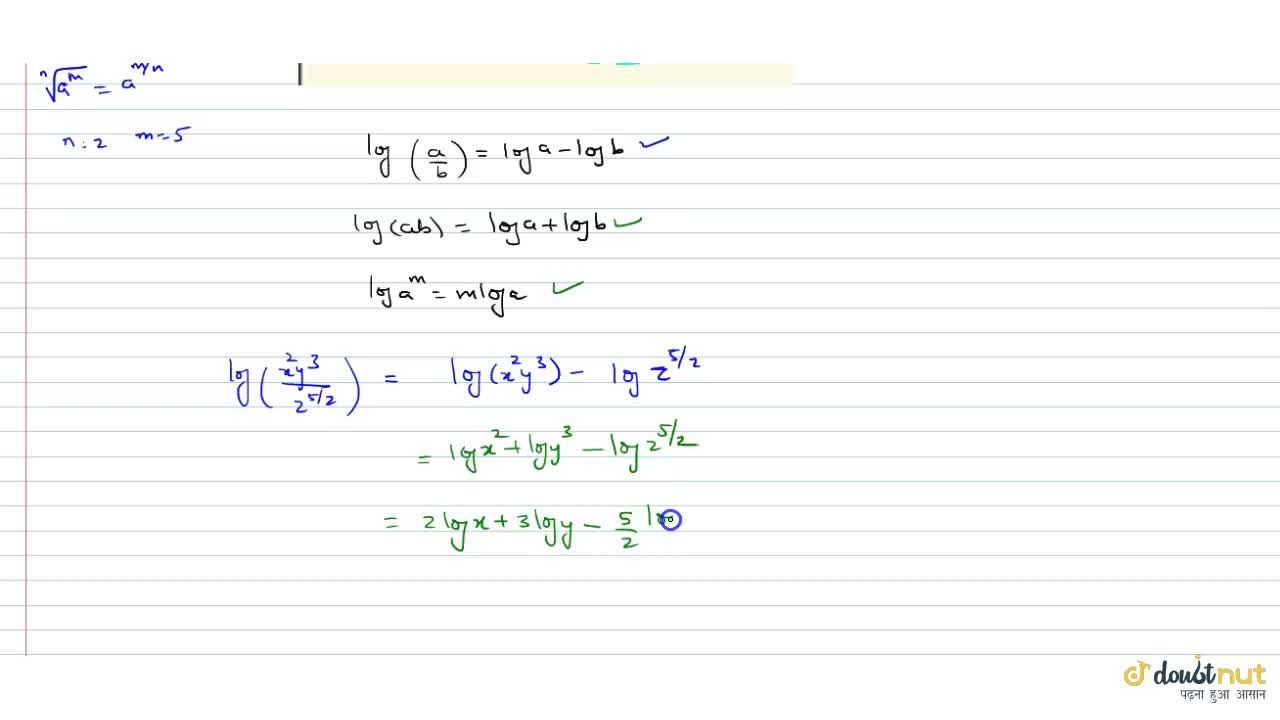



Expand Log X 2 Y 3 Sqrt Z 5




Basic Algebra Activity
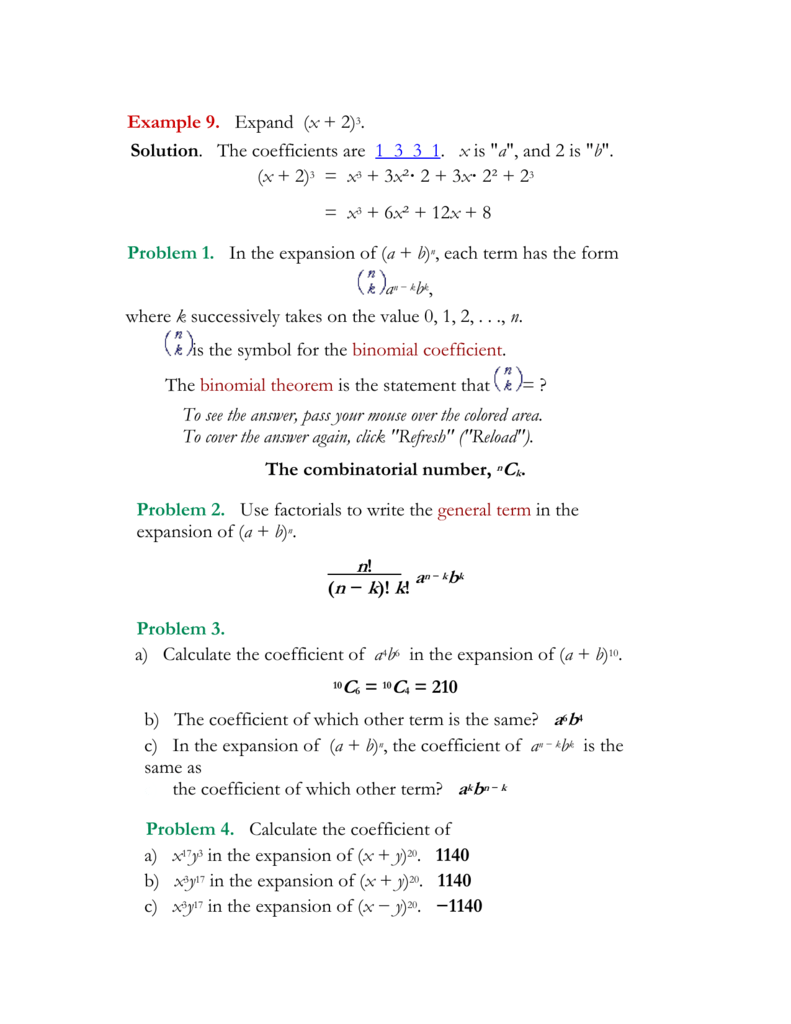



Alg2 March28 The Answers




X Y 2 3 Find The Expansion Of The Following Brainly In



8 4 Pascals Triangle And The Binomial Theorem



E 4 Expand And Condense Logarithms Algebra 3 4



A Lesson In Making Use Of Structure From With Jmccalla1 Experiments In Learning By Doing




Ledoh 98r 15 Expand And Simplify A 3x X 4x 2 Itprospt




Ledoh 98r 15 Expand And Simplify A 3x X 4x 2 Itprospt




Learn Algebraic Identity Of X Y And X Y In 3 Minutes
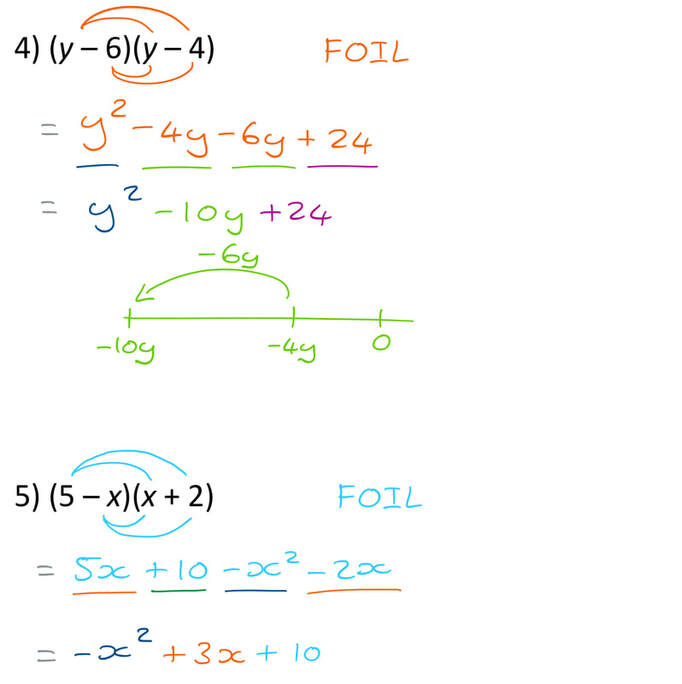



10 Y 3 Best Sale 54 Off Www Nogracias Org
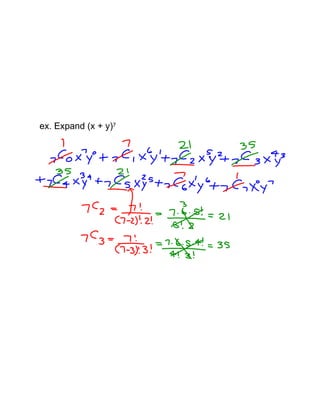



Day 7 Examples U7w14




Expand Y 3x 3 Maths Questions




1 Expand Y 3 X 2 Brainly In



Expand X Y 3 Sarthaks Econnect Largest Online Education Community
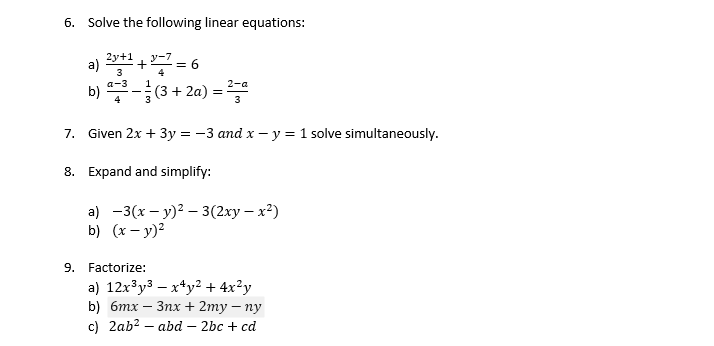



Solved 6 Solve The Following Linear Equations A 2y 1 3 Chegg Com
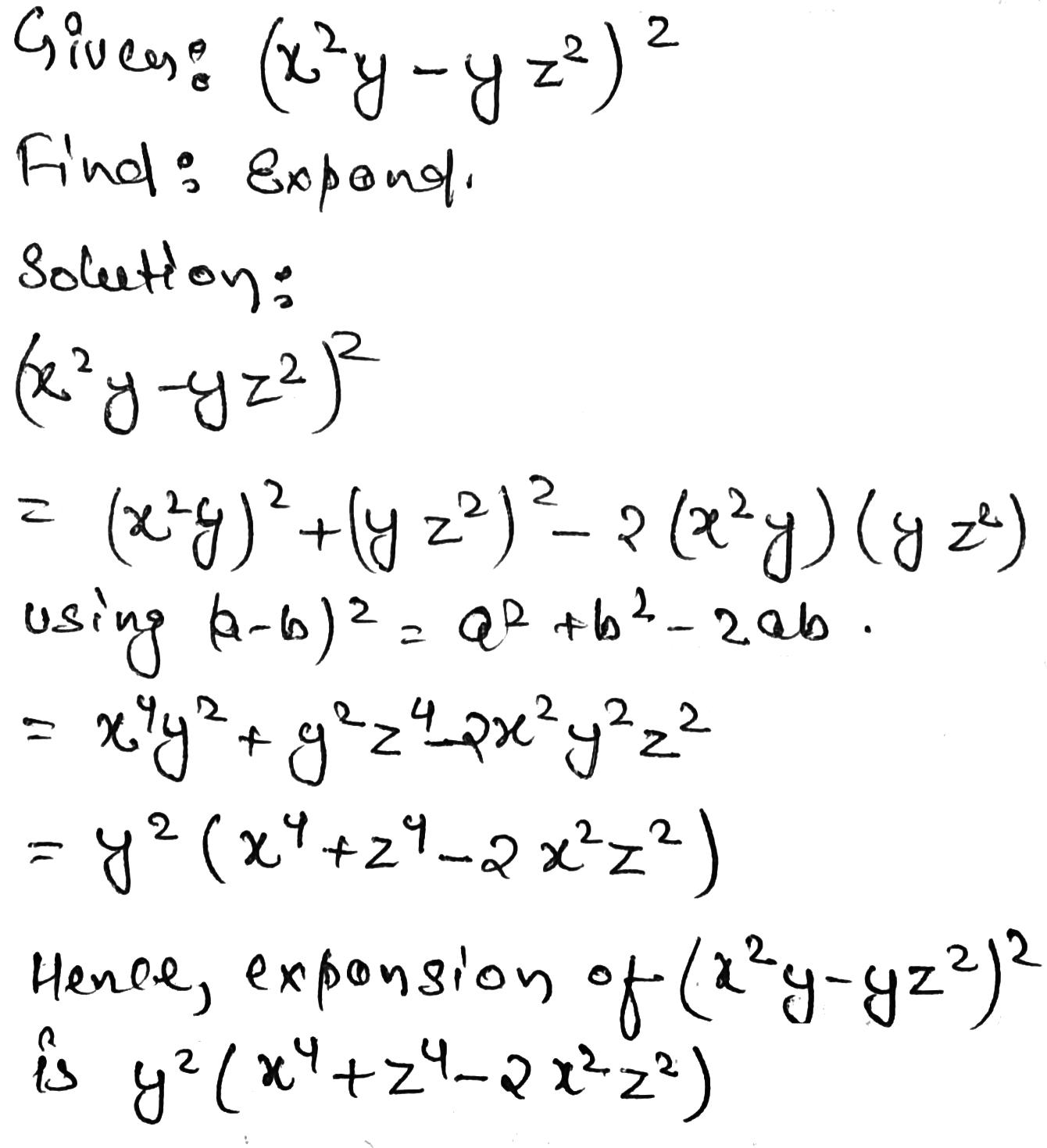



3 Expand Vii X 2 Y Yz 2 2 Snapsolve




Solved 3 Expand And Simplify 12 A X 6 A 6 B Y 3 2 Chegg Com




2 1 Quiz 7 Answers Algebra Introduction Edexcel Gcse Maths Higher Elevise
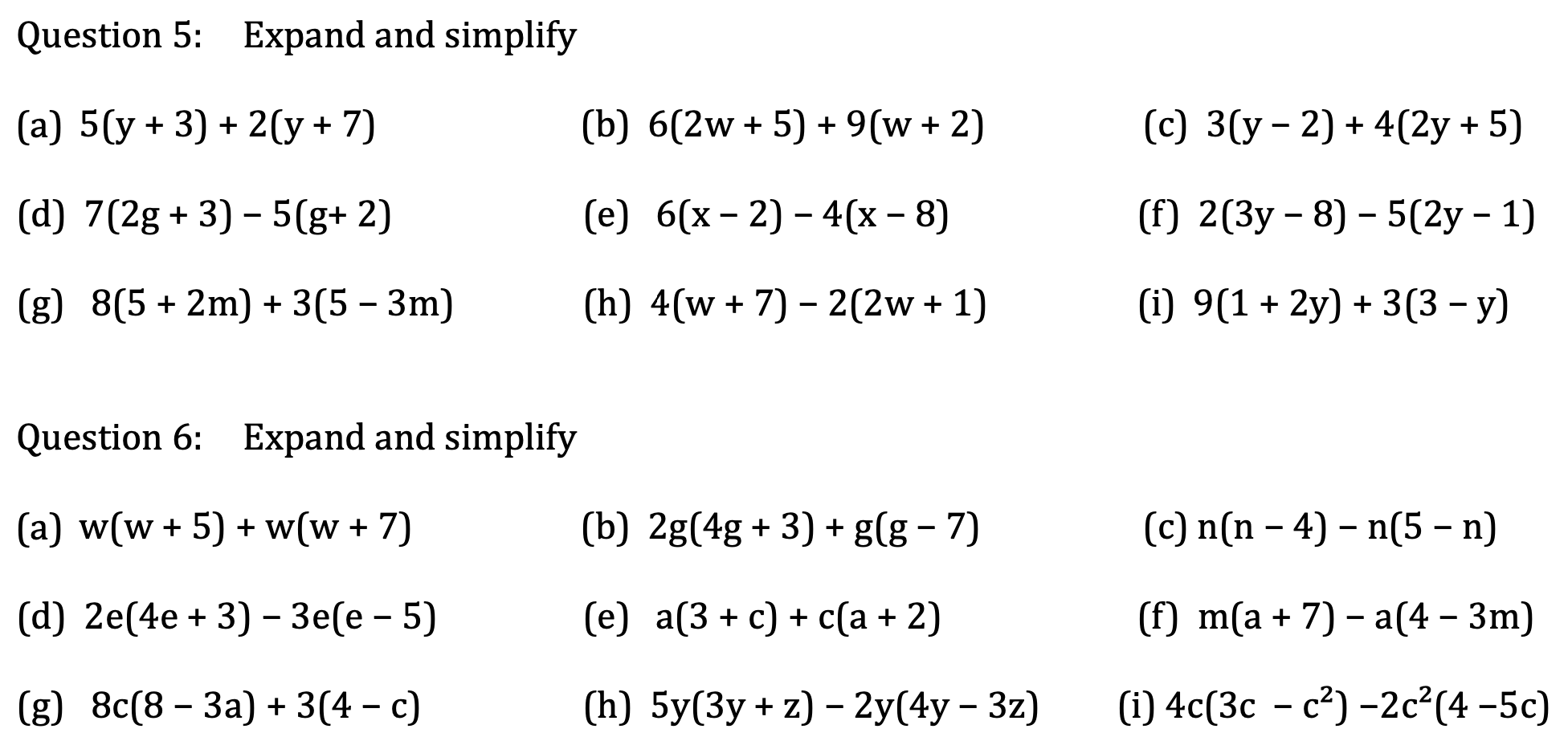



Ks3 Algebra 2 Expanding Brackets Maths With David



Binomial



Answered Use The Laws Of Logarithms To Expand Bartleby
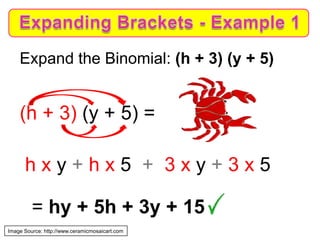



Expanding Binomial Brackets




Expand Each Of The Following Using Suitable Identities I X 2y 4z 2 Ii 2x Y Z Youtube



Pascal S Triangle




Learn Algebraic Identity Of X Y And X Y In 3 Minutes
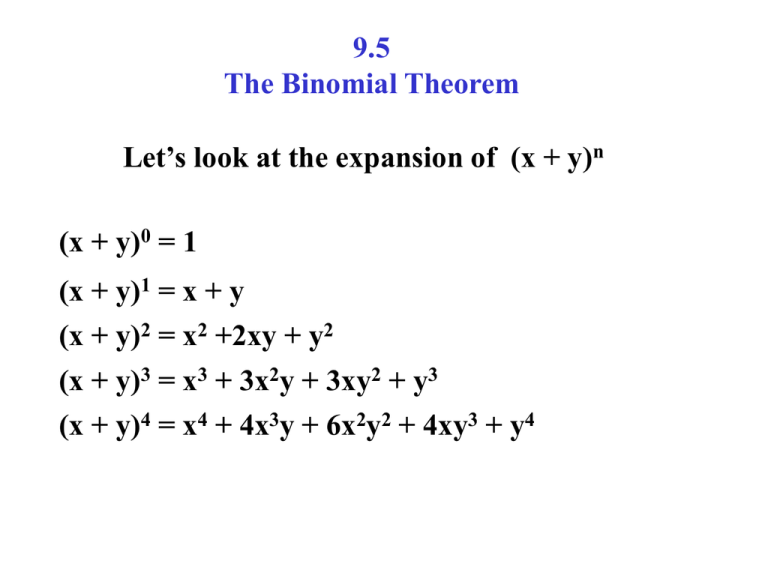



9 5 The Binomial Theorem




8 4 Pascals Triangle And The Binomial Theorem




M I Assignmet 19new Pdf Matrix Mathematics Integral




Expand 1x Y3 3 Maths Questions




Expand X X Y 3 3xy X Y



Expand Tan 1y X About The Point 1 1 Using Taylor S Theorem Up To The Second Degree Terms Sarthaks Econnect Largest Online Education Community




Solved Quiz No 2 Expand The Following 1 X 9 12 2 X Y 9 3 X Y 1s 4 X Y 5 X Y 8



1




How Can We Expand X Y 1 2 Youtube




Expand X Y Sup 6 Sup Hence Evaluate 1 02 Sup 6 Sup To 3d P
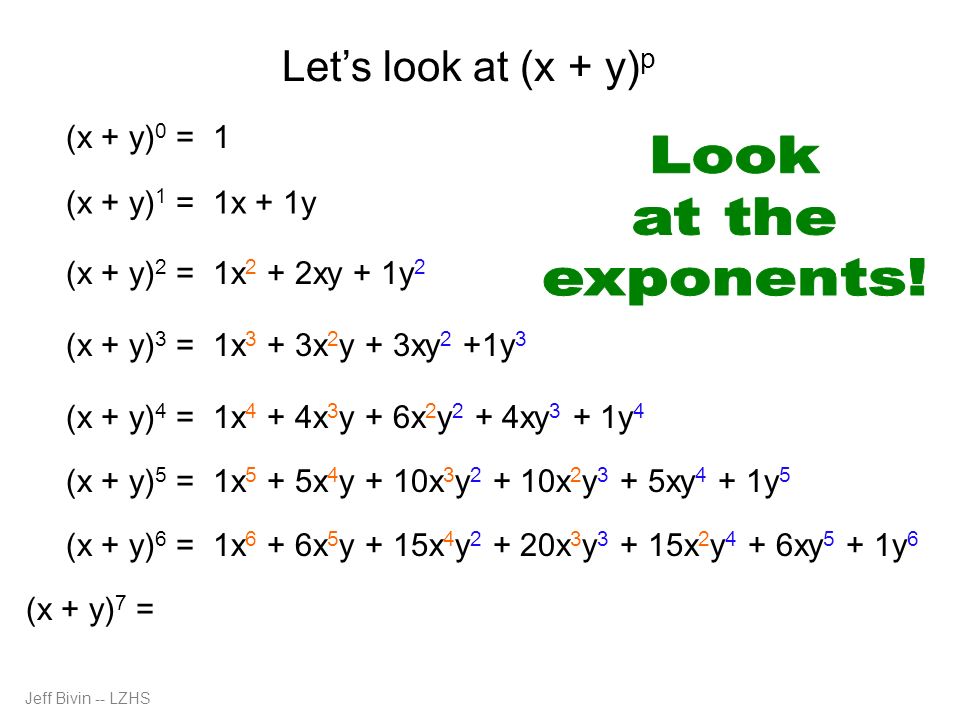



Binomial Expansion And More Ppt Download
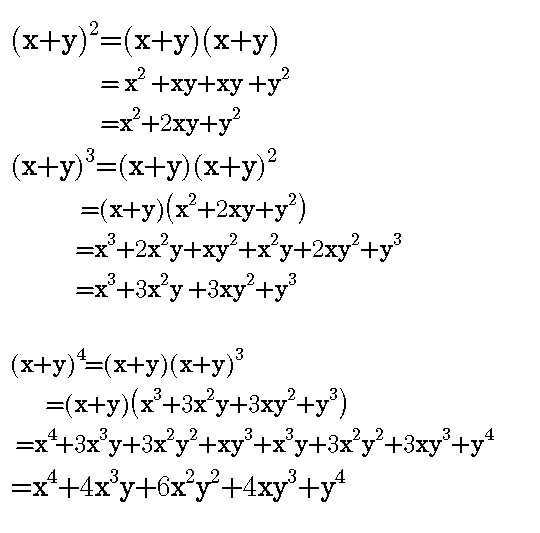



Worked Examples On Binomial Expansion Steemit




Ml Aggarwal Solutions For Class 9 Chapter 3 Expansions Download Free Pdf




Question Expand X 3 Y 2 2 Brainly In




Year 12 C1 Binomial Theorem Task Expand The Following 1 X Y 1 2 X Y 2 3 X Y 3 4 X Y 4 What Do You Notice Powers Of X Start From Ppt Download




Taylor Series Expand X 2y 3y 2 In The Powers Of X 1 And Y 2 Up To 3 Degree Terms Youtube




Pdf G3z3002 Minitest Bash Bash Academia Edu




Sample Practice Exam 11 March Questions Problem Set 6 Mathematics I Ma Autumn 18 Studocu




How To Expand Using The Identity X Y 3 X3 Y3 3x2y 3xy2 Youtube



1




Recall That A Binomial Is A Polynomial That Has Two Terms Which Are




Y9 Algebra Expanding Brackets Maths With David
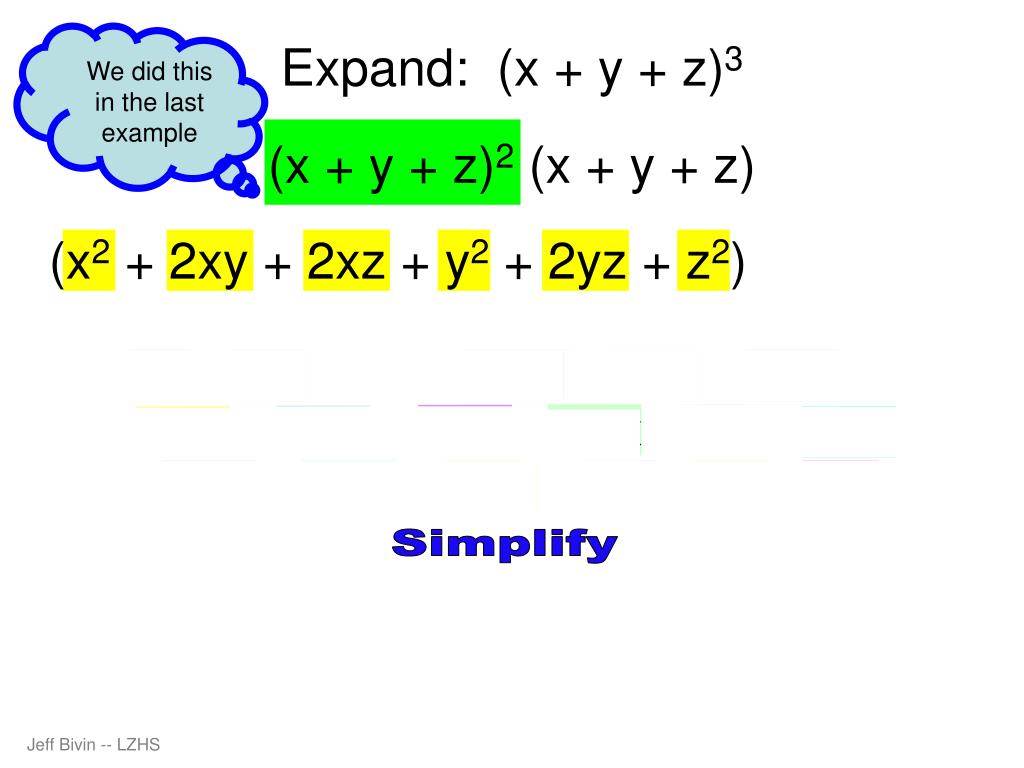



Ppt Binomial Expansion And More Powerpoint Presentation Free Download Id



Expand And Simplify 3 X4 X Y 12 Y 3 X 12 Gauthmath
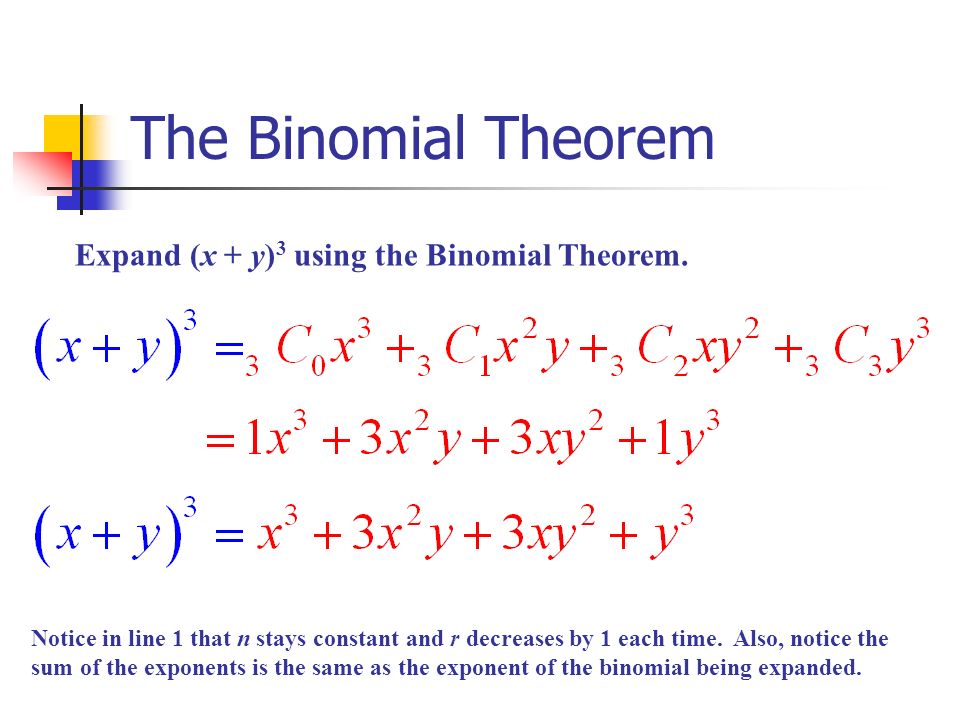



Section 8 5 The Binomial Theorem In This Section You Will Learn Two Techniques For Expanding A Binomial When Raised To A Power The First Method Is Called Ppt Download




Learn Algebraic Identity Of X Y And X Y In 3 Minutes




Expand Each Of The Following Using Suitable Identities 2x 5y 3z 2
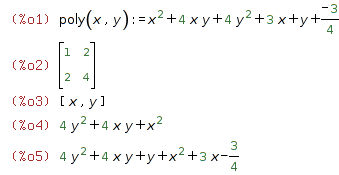



Wxmaxima Html Export



How To Expand X 3y 6 And Apply The Expansion To Evaluate 1 30 5 Correct To Five Decimal Places Quora




3 Dimensional Graphics At L ϒ L 1 L 2 L 3 ϒ 1 ϒ 2 ϒ 3 K Download Scientific Diagram



0 件のコメント:
コメントを投稿